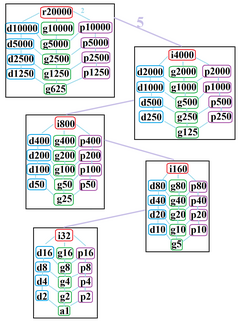
[1][2][3][4][5] A regular myriagon is represented by Schläfli symbol {10,000} and can be constructed as a truncated 5000-gon, t{5000}, or a twice-truncated 2500-gon, tt{2500}, or a thrice-truncated 1250-gon, ttt{1250}, or a four-fold-truncated 625-gon, tttt{625}.

Because 10,000 = 24 × 54, the number of sides is neither a product of distinct Fermat primes nor a power of two.

The regular myriagon has Dih10000 dihedral symmetry, order 20000, represented by 10000 lines of reflection.

These lower symmetries allows degrees of freedom in defining irregular myriagons.

In the novella Flatland, the Chief Circle is assumed to have ten thousand sides, making him a myriagon.