Avrami equation
The Avrami equation describes how solids transform from one phase to another at constant temperature.
It can specifically describe the kinetics of crystallisation, can be applied generally to other changes of phase in materials, like chemical reaction rates, and can even be meaningful in analyses of ecological systems.
The equation was first derived by Johnson, Mehl, Avrami and Kolmogorov (in Russian) in a series of articles published in the Journal of Chemical Physics between 1939 and 1941.
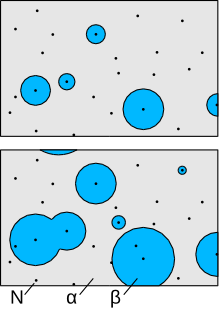
The initial slow rate can be attributed to the time required for a significant number of nuclei of the new phase to form and begin growing.
During the intermediate period the transformation is rapid as the nuclei grow into particles and consume the old phase while nuclei continue to form in the remaining parent phase.
Once the transformation approaches completion, there remains little untransformed material for further nucleation, and the production of new particles begins to slow.
The simplest derivation of the Avrami equation makes a number of significant assumptions and simplifications:[5] If these conditions are met, then a transformation of
, nucleation and growth can only take place in untransformed material.
is one of two parameters in this simple model: the nucleation rate per unit volume, which is assumed to be constant.
Since growth is isotropic, constant and unhindered by previously transformed material, each nucleus will grow into a sphere of radius
is the second of the two parameters in this simple model: the growth velocity of a crystal, which is also assumed constant.
will yield the total extended volume that appears in the time interval: Only a fraction of this extended volume is real; some portion of it lies on previously transformed material and is virtual.
If the transformation follows the Avrami equation, this yields a straight line with slope n and intercept
Thus crystallization takes a time of order i.e., crystallization takes a time that decreases as one over the one-quarter power of the nucleation rate per unit volume,
Typical crystallites grow for some fraction of the crystallization time
, or i.e., the one quarter power of the ratio of the growth velocity to the nucleation rate per unit volume.
Thus the size of the final crystals only depends on this ratio, within this model, and as we should expect, fast growth rates and slow nucleation rates result in large crystals.
The average volume of the crystallites is of order this typical linear size cubed.
Thin films, for example, may be effectively two-dimensional, in which case if nucleation is again uniform the exponent
Originally, n was held to have an integer value between 1 and 4, which reflected the nature of the transformation in question.
In the derivation above, for example, the value of 4 can be said to have contributions from three dimensions of growth and one representing a constant nucleation rate.
Initially, nucleation may be random, and growth unhindered, leading to high values for n (3 or 4).
Once the nucleation sites are consumed, the formation of new particles will cease.
Furthermore, if the distribution of nucleation sites is non-random, then the growth may be restricted to 1 or 2 dimensions.
[7] The Avrami equation was applied in cancer biophysics in two aspects.
First aspect is connected with tumor growth and cancer cells kinetics,[8] which can be described by the sigmoidal curve.
In this context the Avrami function was discussed as an alternative to the widely used Gompertz curve.
This model was applied to clinical data of gastric cancer, and shows that Avrami's constant n is between 4 and 5 which suggest the fractal geometry of carcinogenic dynamics.
[9] Similar findings were published for breast and ovarian cancers, where n=5.3.
[10] The Avrami equation was used by Ivanov et al. to fit multiple times a dataset generated by another model, the so called αDg to а sequence of the upper values of α, always starting from α=0, in order to generate a sequence of values of the Avrami parameter n. This approach was shown effective for a given experimental dataset,[11] see the plot, and the n values obtained follow the general direction predicted by fitting multiple times the α21 model.