In geometry, a decagon (from the Greek δέκα déka and γωνία gonía, "ten angles") is a ten-sided polygon or 10-gon.

[1] Its Schläfli symbol is {10} [2] and can also be constructed as a truncated pentagon, t{5}, a quasiregular decagon alternating two types of edges.

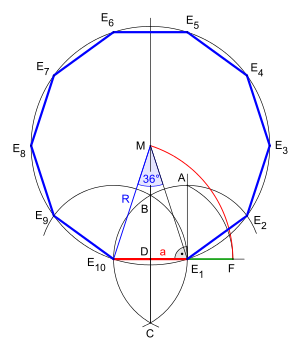
The picture shows a regular decagon with side length

By simple trigonometry, and it can be written algebraically as As 10 = 2 × 5, a power of two times a Fermat prime, it follows that a regular decagon is constructible using compass and straightedge, or by an edge-bisection of a regular pentagon.

[4] An alternative (but similar) method is as follows: Both in the construction with given circumcircle[5] as well as with given side length is the golden ratio dividing a line segment by exterior division the determining construction element.

John Conway labels these by a letter and group order.

Cyclic symmetries in the middle column are labeled as g for their central gyration orders.

Each subgroup symmetry allows one or more degrees of freedom for irregular forms.

These two forms are duals of each other and have half the symmetry order of the regular decagon.

Coxeter states that every zonogon (a 2m-gon whose opposite sides are parallel and of equal length) can be dissected into m(m-1)/2 parallelograms.

[8] In particular this is true for regular polygons with evenly many sides, in which case the parallelograms are all rhombi.

For the regular decagon, m=5, and it can be divided into 10 rhombs, with examples shown below.

This decomposition can be seen as 10 of 80 faces in a Petrie polygon projection plane of the 5-cube.

A skew zig-zag decagon has vertices alternating between two parallel planes.

A regular skew decagon is vertex-transitive with equal edge lengths.

The regular skew decagon is the Petrie polygon for many higher-dimensional polytopes, shown in these orthogonal projections in various Coxeter planes:[9] The number of sides in the Petrie polygon is equal to the Coxeter number, h, for each symmetry family.