Classical control theory
Classical control theory is a branch of control theory that deals with the behavior of dynamical systems with inputs, and how their behavior is modified by feedback, using the Laplace transform as a basic tool to model such systems.
Classical control theory deals with linear time-invariant (LTI) single-input single-output (SISO) systems.
[1] The Laplace transform of the input and output signal of such systems can be calculated.
The transfer function relates the Laplace transform of the input and the output.
In such systems, the open-loop control is termed feedforward and serves to further improve reference tracking performance.
However, time-domain models for systems are frequently modeled using high-order differential equations which can become impossibly difficult for humans to solve and some of which can even become impossible for modern computer systems to solve efficiently.
To counteract this problem, classical control theory uses the Laplace transform to change an Ordinary Differential Equation (ODE) in the time domain into a regular algebraic polynomial in the frequency domain.
Once a given system has been converted into the frequency domain it can be manipulated with greater ease.
[2] Classical control theory uses the Laplace transform to model the systems and signals.
The Laplace transform is a frequency-domain approach for continuous time signals irrespective of whether the system is stable or unstable.
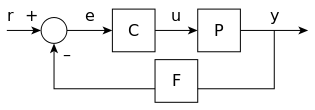
The Laplace transform of a function f(t), defined for all real numbers t ≥ 0, is the function F(s), which is a unilateral transform defined by where s is a complex number frequency parameter A common feedback control architecture is the servo loop, in which the output of the system y(t) is measured using a sensor F and subtracted from the reference value r(t) to form the servo error e. The controller C then uses the servo error e to adjust the input u to the plant (system being controlled) P in order to drive the output of the plant toward the reference.
For some distributed parameter systems the vectors may be infinite-dimensional (typically functions).
If we assume the controller C, the plant P, and the sensor F are linear and time-invariant (i.e., elements of their transfer function C(s), P(s), and F(s) do not depend on time), the systems above can be analysed using the Laplace transform on the variables.
, a PID controller has the general form The desired closed loop dynamics is obtained by adjusting the three parameters
The integral term permits the rejection of a step disturbance (often a striking specification in process control).
Applying Laplace transformation results in the transformed PID controller equation with the PID controller transfer function There exists a nice example of the closed-loop system discussed above.
If we take PID controller transfer function in series form 1st order filter in feedback loop linear actuator with filtered input and insert all this into expression for closed-loop transfer function
For practical PID controllers, a pure differentiator is neither physically realisable nor desirable[3] due to amplification of noise and resonant modes in the system.
Therefore, a phase-lead compensator type approach is used instead, or a differentiator with low-pass roll-off.
More advanced tools include Bode integrals to assess performance limitations and trade-offs, and describing functions to analyze nonlinearities in the frequency domain.