Empirical evidence for the spherical shape of Earth

The roughly spherical shape of Earth can be empirically evidenced by many different types of observation, ranging from ground level, flight, or orbit.

On a completely flat Earth without obstructions (mountains, hills, valleys or volcanos), the ground itself would never obscure distant objects.

Refraction can give the impression that Earth's surface is flat, curved more convexly than it is, or even that it is concave (this is what happened in various trials of the Bedford Level experiment).

This is often seen at sunset, when the Sun's shape is distorted, but has also been photographed happening to ships, and has caused the city of Chicago to appear normally, upside down, and broken into pieces from across Lake Michigan (from where it is normally below the horizon).

The only shape that casts a round shadow no matter which direction it is pointed is a sphere, and the ancient Greeks deduced that this must mean Earth is spherical.

This effect of geometry means that, when viewed from a high mountain, flat ground or ocean blocks less than a hemisphere of the sky.

Using observations of the star Canopus at Rhodes (in Greece) and Alexandria (in Egypt) and the distance between them, the Ancient Greek philosopher Posidonius used this technique to calculate the circumference of the planet to within perhaps 4% of the correct value.

The Spanish Muslim astronomer Ibn Rushd went to Marrakesh (in Morocco) to observe the same star in 1153, as it was invisible in his native Córdoba, Al-Andalus.

At the South Pole, a completely different set of constellations are visible during the six months of continuous night, including Crux, and Centaurus.

The direction any intermediate spot on Earth is facing can also be calculated by measuring the angles of the fixed stars and determining how much of the sky is visible.

In locations just outside the polar circles, there are so-called "white nights" in the middle of summer, in which the sun is never more than a few degrees below the horizon in June such that a bright twilight persists from sunset to sunrise.

[14] Longer twilights are observed at higher latitudes (near the poles) due to a shallower angle of the Sun's apparent movement compared to the horizon.

On a non-curved, flat landmass it would only take seconds, due to minuscule ratio (compare ~45 meters / 150 feet of a 14-story building to intercontinental distances).

If such a phenomenon were caused by a prismatic property of atmosphere in a flat world, with a relatively small source of light revolving around Earth (as in later, 1800's-dated, maps of Flat Earth), it would contradict with one's ability to see a proper panorama of starry sky at a time at night, rather than a small yet distorted, "stretched" patch of it.

Though modern telecommunications and timekeeping were not available, he was able to make sure the measurements happened at the same time by having them taken when the Sun was highest in the sky (local noon) at both locations.

(A full range of angles will be observed if the north and south poles are included, and the day chosen is either the autumnal or spring equinox.)

Explorers, government researchers, commercial pilots, and tourists have been to Antarctica and found that it is not a large ring that encircles the entirety of Earth, but actually a roughly disk-shaped continent smaller than South America but larger than Australia, with an interior that can in fact be traversed in order to take a shorter path from, for example, the tip of South America to Australia than would be possible on a disk.

The resulting discontinuities are sometimes reflected directly in local roads, which have kinks where the grid cannot follow completely straight lines.

The laws of gravity, chemistry, and physics that explain the formation and rounding of Earth are well-tested through experiment, and applied successfully to many engineering tasks.

[13] Such a conjecture would also leave open for explanation the tides seen in Earth's oceans, which are conventionally explained by the gravity exerted by the Sun and Moon.

The Earth would also quickly approach light-speed in this scenario because the pull of gravity would increase by -9.8m/s, each second (as the formula for gravitational acceleration is measured in m/s2).

The mathematics of navigation using Global Positioning System (GPS) satellites assumes that they are moving in known orbits around an approximately spherical surface.

The accuracy of GPS navigation in determining latitude and longitude and the way these numbers map onto locations on the ground show that these assumptions are correct.

Equatorial mounts allow astronomers to point telescopes at the same celestial object for longer times while compensating for Earth's rotation in an easy way.

For example, the towers of the Humber Bridge, although both vertical with respect to gravity, are 36 mm (1.4 inches) farther apart at the top than the bottom due to Earth's curvature.

[29] Low-flying planes and commercial airliners do not necessarily fly high enough to make this obvious, especially when passenger windows narrow the field of view or clouds or terrain reduce the effective height from the visible surface.

[30][31] Trying to measure the curvature of the horizon by taking a picture is complicated by the fact that both windows and camera lenses can produce distorted images depending on the angle used.

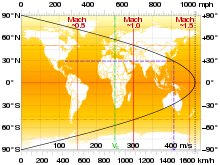
[33] Because the speed of the Sun's shadow is slower in polar regions (due to the steeper angle), even a subsonic aircraft can overtake the sunset when flying at high latitudes.

One photographer used a roughly circular route around the North Pole to take pictures of 24 sunsets in the same 24-hour period, pausing westward progress in each time zone to let the shadow of the Sun catch up.

[citation needed] In the documentary Behind the Curve, Bob Knodel uses a ring-laser gyroscope to attempt to prove that the earth does not rotate.